Archimedes’ Principle

How to use this exercise?

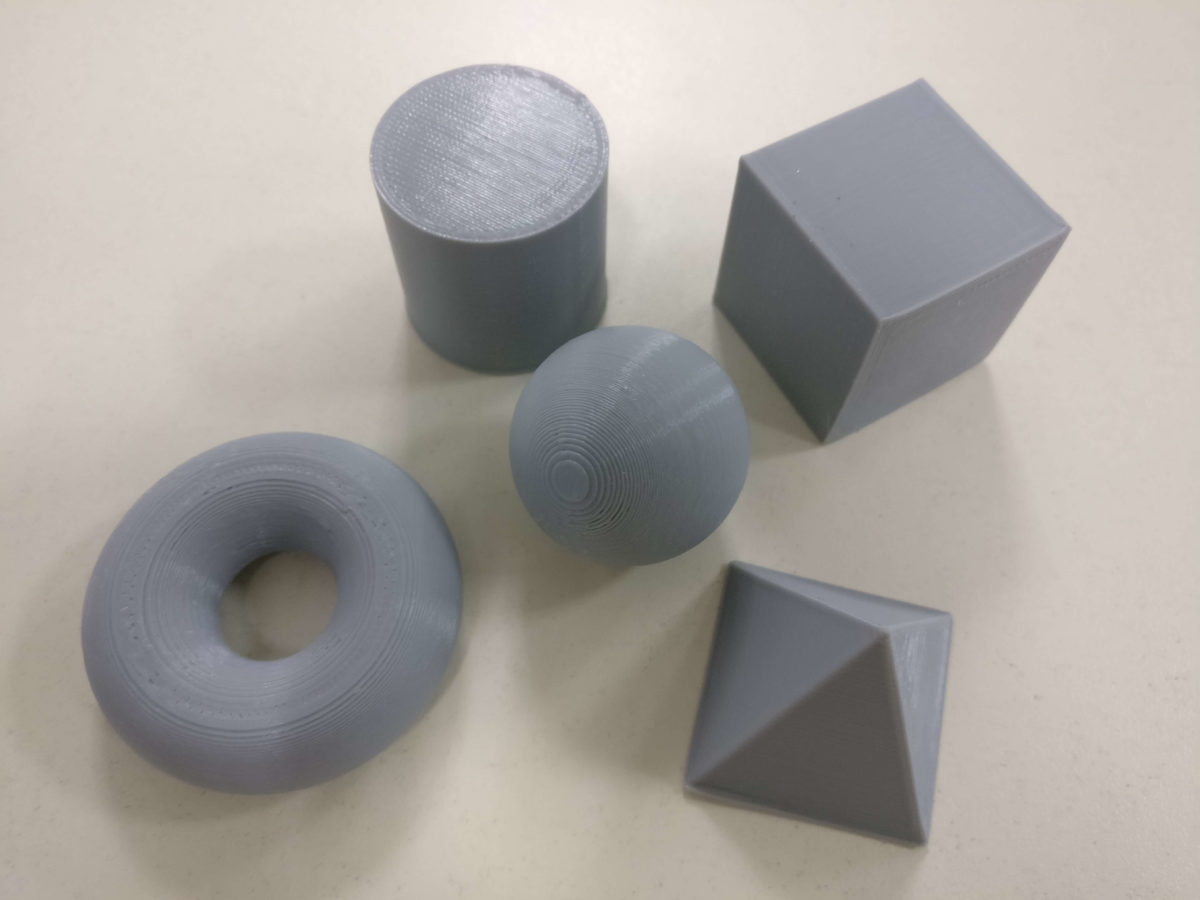
Students will be able to prove the Archimedes’ Principle by immersing different pieces in a measuring cylinder.

Number of pieces of this exercise

5

General description

The idea is to print pieces with different basic geometries, such as cylinders, spheres, cubes, pyramids, etc. Students will have to calculate the volume of these pieces and then immerse them in a measuring cylinder filled with water. They will have to check how much water the piece is displacing and verify that is the same as the volume of the piece.

It is advisable to print the pieces with 100% of infill, so that they are completely immerse in the water.

Example printing a cube:

Cube volume: a*a*a = 2cm*2cm*2cm = 8cm3

Displaced volume in the measuring cylinder:
First of all, students will have to measure the internal diameter of the measuring cylinder. Let say this diameter is 3 cm.
If students immerse the cube inside the measuring cylinder filled with water, they will able to see that the displaced height is approximately 1.15cm.
So, they will have to check that the displaced volume is equal to the volume of the cube:

Pi*R2*Δh = a*a*a  3.14*1.52*1.15 = 8.1 ≈ 8

Additional Material

Models used:

License Info

You are free to:

Share — copy and redistribute the material in any medium or format
Adapt — remix, transform, and build upon the material
for any purpose, even commercially.

Under the following terms:

Attribution — You must give appropriate credit, provide a link to the license, and indicate if changes were made. You may do so in any reasonable manner, but not in any way that suggests the licensor endorses you or your use.
No additional restrictions — You may not apply legal terms or technological measures that legally restrict others from doing anything the license permits.